Author:
(1) Sima Mehri, Farzanegan High School.
Table of Links
Abstract and 1 Introduction and Description of Results
2. Proof of Results and References
2. Proof of Results
In the proof of both theorems, we use induction.
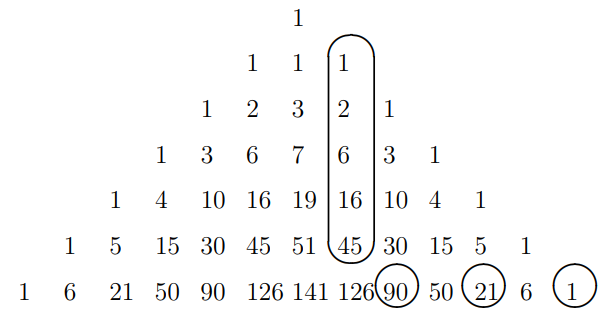
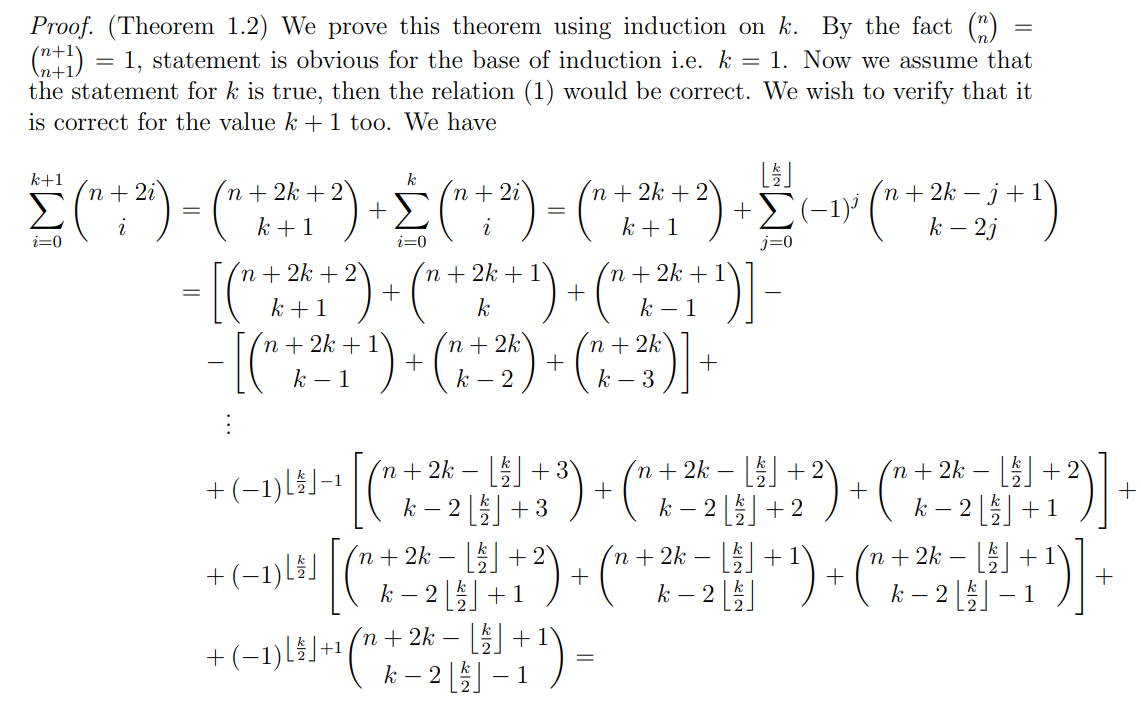
using properties of Pascal triangle, we get
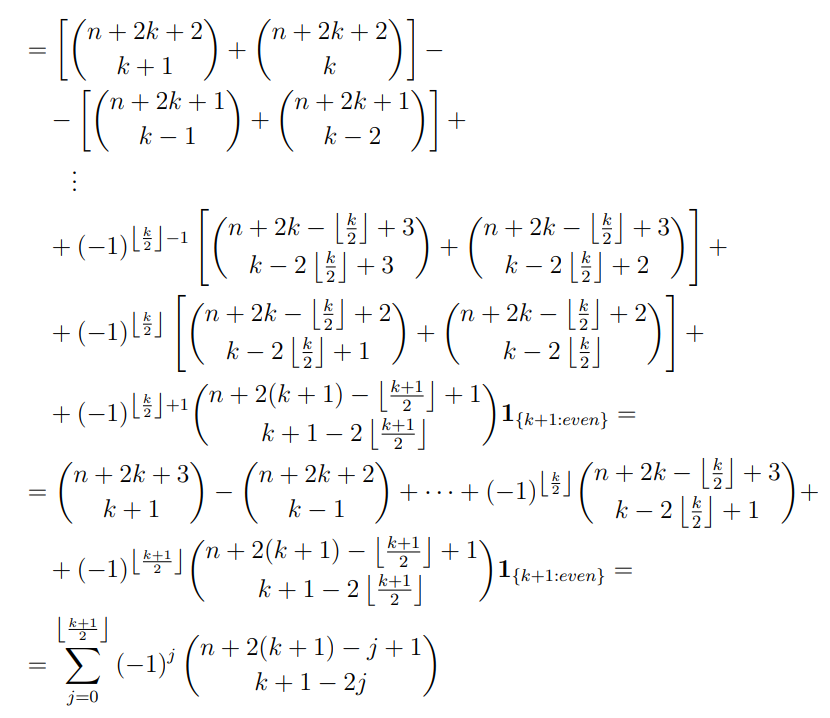
The statement for k + 1 is also true, and the proof is completed.
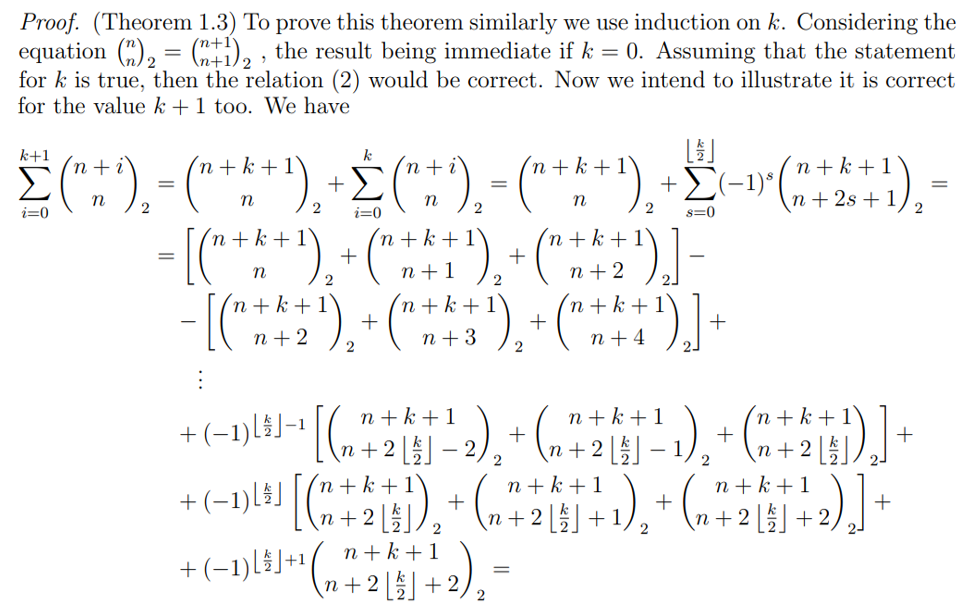
using properties of the trinomial coefficients, we get
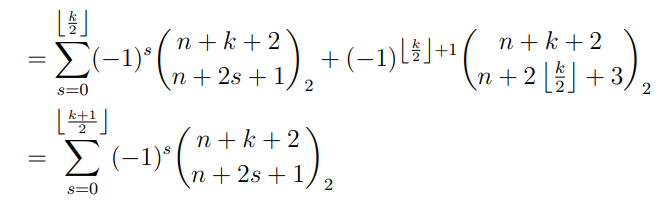
The statement for k + 1 is also true, and the proof is completed.
The hockey stick theorem in the trinomial triangles has been proved. This theorem can be translated in Pascal pyramid as follows :
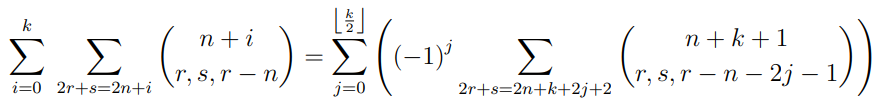
Other similar theorems might be obtained for Pascal’s four dimensional and even n-dimensional pyramid.
References
1] G. Andrews, Euler’s ’Exemplum Memorabile Inductionis Fallacis’ and Trinomial Coefficients J. Amer. Math. Soc. 3 (1990), 653-669.
[2] P. Hilton and J. Pedersen, Looking into Pascal Triangle, Combinatorics, Arithmetic and Geometry Mathematics Magazine, Vol. 60, No. 5 (Dec., 1987), 305-316.
[3] Eric W.Weisstein, Trinomial Coefficient From MathWorld–A Wolfram Web Resource. http://mathworld.wolfram.com/TrinomialTriangle.html
[4] Eric W.Weisstein, Trinomial Triangle From MathWorld–A Wolfram Web Resource. http://mathworld.wolfram.com/TrinomialTriangle.html
This paper is available on arxiv under CC BY 4.0 DEED license.

